What Are Stationary Waves?
Stationary wave also referred to as standing wave is a wave which oscillates in time but whose peak amplitude profiles does not move in space. The peak amplitude of the wave oscillations at any point in space is constant with time, and the oscillations at different points throughout the wave are in phase. The phenomenon is the result of interference between two waves travelling in opposite directions; their energies are either added together or cancelled out. This interference occurs in such a manner that specific points along the medium appear to be standing still.
Standing waves were first noticed by Michael Faraday in 1831. On a stationary wave, the locations at which the absolute value of the amplitude is minimum is referred to as nodes and the locations where the absolute value of the amplitude is maximum are referred to as antinodes. All the particles in a given segment vibrate in phase but in opposite phase relative to the particles in the adjacent segment.
In a progressive wave, the particles at the nodes are permanently at rest but other particles attain their position of momentary rest simultaneously. All the particles attain their own maximum velocity at the same time when they pass through their mean positions. In the case of a longitudinal stationary wave, the variation of density is different at different points being maximum at the nodes and zero at the antinodes.
The most common cause of standing waves is the phenomenon of resonance in which standing waves occur inside a resonator due to interference between waves reflected back and forth at the resonator’s resonant frequency.
One common example of standing waves is two people shaking either end of a jump rope. If they shake in sync the rope can form a regular pattern of waves oscillating up and down, with stationary points along the rope where the rope is almost still (nodes) and points where the arc of the rope is maximum (antinodes). Standing waves are also observed in optical media such as optical waveguides and optical cavities.
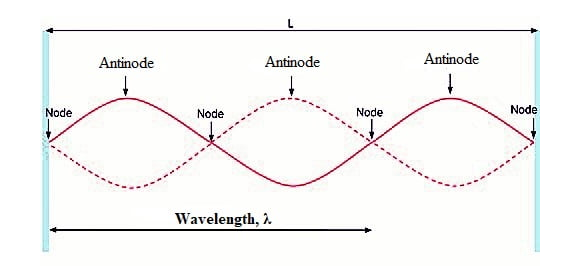
Characteristics Of Stationary Waves
- A stationary wave also referred to as standing wave remains in a constant position.
- The energy is not propagated onwards because each particle possesses its own characteristic vibration.
- The particles of the medium at nodes are permanently at rest but other particles attain their position of momentary rest simultaneously.
- The amplitude of vibration of the particles changes from zero at nodes to maximum at antinodes.
- All the particles in a given segment vibrate in phase but in opposite phase relative to the particles in the adjacent segment.
- All the particles attain their own maximum velocity at the same time when they pass through their mean positions.
- Stationary waves do not undergo forward motion and due to its crests and troughs blink at the initial position.
- In stationary wave, the distance between three successive nodes or anti-nodes is referred to as wavelength.
- The wavelength of stationary wave is denoted as twice the distance between adjacent nodes (nλ=2L, where n=1, 2, 3…).
- All particles oscillate at the same frequency but the particles at nodes do not.
- In a longitudinal stationary wave, the variation of density is different at different points being maximum at the nodes and zero at the antinodes.
- The equation of a stationary wave is, y= A sin 2π/λ (vt)
Also Read: Difference Between Primary And Seismic Waves
What Are Progressive Waves?
Progressive wave also referred to as travelling wave, is a wave which travels continuously in medium in the same direction without the change in its amplitude. They are those waves having visible disturbances meaning the vibrations can be seen moving throughout the material. The disturbance produced in the medium travels onward, it being handed over from one particle to the next. Each particle executes the same types of vibration as the preceding one, though not at the same time. The amplitude of each particle is the same but the phase changes continuously.
In a progressive wave, no particle is permanently at rest. Different particles attain the state of momentary rest at different instants. All the particles attain the same maximum velocity when they pass through their mean positions. In the case of a longitudinal progressive wave, all the parts of the medium undergo similar variation of density one after the other. At every point there will be a density variation. Examples of progressive waves are sound waves, water waves, etc.
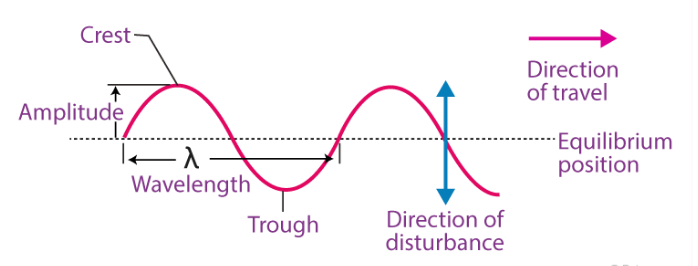
Characteristics Of Progressive Waves
- A progressive wave also referred to as travelling wave moves away from the source. It moves relative to a coordinate system in a fluid.
- The energy is transmitted from one point to another due to movement of vibration within the molecules of the wave.
- No particle of the medium is permanently at rest. Different particles attain the state of momentary rest at different instants.
- The amplitude of vibration of each particle is the same throughout but there is significant variation in phase.
- Each particle executes the same type of vibration as the preceding one, though not at the same time.
- All the wave particles attain the same maximum velocity when they pass through their mean positions.
- In progressive wave the wave undergoes forward motion and thus the crests and troughs are also in motion.
- In progressive wave, the distance between successive crests of a transverse wave and distance between compression and rarefaction is referred to as wavelength.
- The wavelength of a progressive wave is simply equal to the distance between the particles at the same phase (nλ=L, where n=1, 2, 3..).
- Particles oscillate at the same frequency.
- In a longitudinal progressive wave, all the parts of the medium undergo similar variation of density one after the other. At every point, there will be a density variation.
- The equation of a progressive wave is, y= A sin 2π/λ (vt-x)
Also Read: Difference Between Longitudinal And Transverse Waves
Difference Between Stationary And Progressive Waves In Tabular Form
| BASIS OF COMPARISON | STATIONARY WAVES | PROGRESSIVE WAVES |
| Description | A stationary wave also referred to as standing wave remains in a constant position. | A progressive wave also referred to as travelling wave moves away from the source. It moves relative to a coordinate system in a fluid. |
| Energy | The energy is not propagated onwards because each particle possesses its own characteristic vibration. | The energy is transmitted from one point to another due to movement of vibration within the molecules of the wave. |
| Particle At Nodes | The particles of the medium at nodes are permanently at rest but other particles attain their position of momentary rest simultaneously. | No particle of the medium is permanently at rest. Different particles attain the state of momentary rest at different instants. |
| Amplitude Of Vibration | The amplitude of vibration of the particles changes from zero at nodes to maximum at antinodes. | The amplitude of vibration of each particle is the same throughout but there is significant variation in phase. |
| Particle Vibration | All the particles in a given segment vibrate in phase but in opposite phase relative to the particles in the adjacent segment. | Each particle executes the same type of vibration as the preceding one, though not at the same time. |
| Particle Velocity | All the particles attain their own maximum velocity at the same time when they pass through their mean positions. | All the wave particles attain the same maximum velocity when they pass through their mean positions. |
| Forward Motion | Stationary waves do not undergo forward motion and due to its crests and troughs blink at the initial position. | In progressive wave the wave undergoes forward motion and thus the crests and troughs are also in motion. |
| Wavelength | In stationary wave, the distance between three successive nodes or anti-nodes is referred to as wavelength. | In progressive wave, the distance between successive crests of a transverse wave and distance between compression and rarefaction is referred to as wavelength. |
| Wavelength Equation | The wavelength of stationary wave is denoted as twice the distance between adjacent nodes (nλ=2L, where n=1, 2, 3…). | The wavelength of a progressive wave is simply equal to the distance between the particles at the same phase (nλ=L, where n=1, 2, 3..). |
| Particle Oscillation | All particles oscillate at the same frequency but the particles at nodes do not. | Particles oscillate at the same frequency. |
| Variation Of Density | In a longitudinal stationary wave, the variation of density is different at different points being maximum at the nodes and zero at the antinodes. | In a longitudinal progressive wave, all the parts of the medium undergo similar variation of density one after the other. At every point, there will be a density variation. |
| Equation | The equation of a stationary wave is, y= A sin 2π/λ (vt) | The equation of a progressive wave is, y= A sin 2π/λ (vt-x) |
Also Read: Difference Between Mechanical And Electromagnetic Waves